Music Home | Home Page 

Music Definitions
 

A combination of three or more tones played together.
 

chromatic

A scale that progresses by half-tones, i.e. uses all the sharps and flats.
 

dynamic range

The range of sound volume between the softest perceptible and the loudest possible.  There are many occasions this comes into play.  Recording media has a dynamic range because background noise will mask the sound below a certain level, and the sound will become distorted beyond a maximum volume.  The dynamic range of each medium (e.g. record, tape, cd) differs due to the physical differences inherent to that medium.  The dynamic range of each type of instrument is also different, based on limitations in the ability to produce a softer or louder tone on that instrument.  The acoustic environment involved will change the dynamic range characteristics of the human auditory system as well.

In terms of human hearing, the enormous range in our ability to detect even the softest whispers, while not being overwhelmed by the intensity of a low-flying jet aircraft passing overhead is vastly increased due to the logarithmic nature of how our system processes sounds.  It takes a sound that is four times louder than other before it will seem to be only twice as loud, because we decode exponential increases in volume linearly.

exponential curve

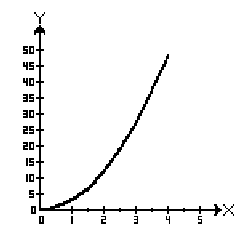If the reader is not familiar with equations that express various lines and curves, or the graphing techniques used to depict them, it is suggested that the entry on linear equations be read first as a baseline comparison for understanding how an exponential curve differs.

Consider an equation such as y = 3x².  In this example, if x = 2 then y = 3 X 2² = 3 X 2 X 2 = 12.  But if x = 3 then y = 3 X 3² = 3 X 3 X 3 = 27.  Notice that in the first case the output is 6 times the input, but in the second case it is 9 times the input.  This means that the slope is changing, and the equation is therefore not linear.  Because the 2 in the expression x² is called an exponent, the resulting graph for this equation is called an exponential curve.  This equation is plotted in the figure.  Note that the exponent can be other positive rational numbers besides 2 (x times x) –– it can be a 3 (x times x times x), or 3/2 (the square root of x times x times x), or 5/3 (the cube root of x times x times x times x times x).

Fourier

Joseph Fourier discovered that complex periodic waveforms could be broken down into their single-frequency components, each having its own amplitude value, and could be expressed as a series of additive terms that are based on the sine function.  This weighted sum of individual sinusoids is called a Fourier series.

frequency

Sound waves are the result of some form of vibration that causes air particles in the vicinity to move back and forth, causing other adjacent air particles to do the same, and eventually spreading out in all directions like the ripples seen when a stone is cast in the middle of a calm pond.  The number of times a sound wave completes its complete forward and backward journey in one second is its frequency, and is measured in Hertz (Hz) or "cycles per second".  Sounds are heard when these sound waves intercept a human or animal eardrum and cause it to then vibrate at the same frequency, which the brain can then decode into what you "hear".  See harmonic for some background on the makeup of musical tones and how frequency relates to pitch.

harmonic

Sounds can be very complex, consisting of many different frequencies, but pure musical tones (as opposed to chords, which are combinations of pure tones) have a basic or "root" frequency upon which other higher tones are added.  This root frequency, or fundamental, determines the pitch of the note you are hearing.   The more pleasant sounding tones are those where most of the higher tones that are mixed in are some simple multiple of this fundamental frequency, and are then said to be a harmonic of it.  For instance, if the root is 440 Hz, then some examples of its harmonics would be 880 Hz (2 times), 1320 Hz (3 times), and 660 Hz (3/2 times).

linear equations

An algebraic equation is simply a mathematical formula where letters, either variables or constants, take the place of actual numbers temporarily.  A constant is a known value that may change depending on the exact equation we are dealing with, and is thus shown by a letter "placeholder" in a more generalized version of the equation, but the real value can be "plugged into" the equation at any time.  A variable, however, is generally an unknown, and if there is only one within a single equation, then one can solve for it (i.e. isolate it and learn its value by applying certain basic rules of manipulation).  If there are more than two variables in a single equation, then additional equations are required in order to solve it.  But if there are exactly two variables, one can look at it as a black box with an input and an output.  The input is often chosen to be represented by an "x , in which case the output is typically denoted as a "y".  When graphing such an equation, or function, the input is then shown along the horizontal axis (x-axis) and the output is shown on a vertical axis (y-axis), as shown in the figure.  As one chooses arbitrary values for x (note the use of lower case here to distinguish it from an "X" which will be used to signify multiplication here), and solves for "y", the pair of values can be located on the graph by moving along the x-axis to the value chosen for "x", and then up the y-axis to " y". This location can be marked with a dot, and after solving for multiple values of "x", one can then connect the dots to see if the equation defines a straight line or an identifiable curve (e.g. a circle, an ellipse, a sine wave, or a parabola).  If it does, then all other values of "x" can be extrapolated, and the output value for any input value can be determined from the graph without actually plugging the value in for "x" and calculating "y".  An example will make this clearer.

A linear function, for instance, takes the form of y = mx + b, where m and b are both constants.   Thus the equation y = 3x + 5 is linear.  If you put in x = 10, then you get y = 3 X 10 + 5 = 35 out.  And likewise, for x = 3 then y = 3 X 3 + 5 = 14.  This is shown in the figure below where the two points are then connected by a black line that extends beyond them to show all values of "x" within the range of the chart.  Notice that if we didn't know it was linear from the form of the equation, we would soon see this after adding more x,y pairs.  We can also now mention that the "b" in the original equation, which is a 5 in our example, simply indicates where the line will cross the y-axis –– and this occurs when x = 0.  In this case, for x = 0 then y = 3x + 5 = 3 X 0 + 5 = 5, so you will see that the line crosses the y-axis at y = 5.  The "m" in the original equation is called the "slope" of the line.  Since we chose "m" to be 3 in our example, this means that if x = 5 then y = 3 X 5 + 5 = 20, and if x = 7 that makes y = 3 X 7 + 5 = 26.  Notice that the difference in output values for these points is 14 - 11 = 3, which is 3 times the difference in input values of 3 - 2 = 1.  This ratio of change (symbolized by the greek letter Δ) in output over change in input will be seen to be the same no matter which values of "x " we choose, and this constant "slope" is an important and distinguishing feature of linear equations.  By the way, a slope of m = 6 instead of m = 3 would result in the red trace shown in the figure.

logarithm

The concept of logarithms is based on determining what exponent of some number will equal some other desired number.  Except for natural logarithms, which use "ln" instead of "log" in their equations, log means to the base ten unless otherwise specified.  And that means that log25 is resolved by asking what number is x such that 25 = 10^x??  If another base is intended, such as 25 = 2^x, then it is written as log₂25.

Now, we can easily see that the log of 100 is 2 because 10² = 100, and the log of 1000 is 3 since 10 X 10 X 10 = 10³ = 1000.  But since the solution is not so readily apparent for numbers other than 10 and 100 and 1000, and there is no simple way to calculate a logarithm, charts were used for years until calculators became commonplace.

Going a step further, the log of 25, when rounded, would be written as log25 = 1.4, which means 25 = 10^1.4.  If it seems strange to think about multipling 10 by itself 1.4 times, this is the same as 10^14/10 = 10^7/5 which simply means taking the fifth root of 10⁷.  Try it!!

One place where logarithms are handy is when solving exponential equations like y = 2^3/2 X 3².  This could be solved by √(2³) X 3² = √8 X 9 = 18√2 ≈ 25.46, or by taking the logarithm of both sides as follows:

log y = log 2^3/2 + log3²

log y = 3/2 X log 2 + 2 X log 3

log y ≈ 3/2 X 2.828 + 2 X 0.4771

log y ≈ 1.406

y ≈ 25.46

The benefit lies in several areas.  First, note that instead of having to multiply two exponential factors, the exponents come down and become simple factors...much easier to multiply.  But more importantly, notice that the logarithms of these original factors are not multiplied, but are added!!  Exponents become simple multiplication and multiplication becomes addition - basically taking everything down one level of complexity.  As a note of explanation, y is found in the last line by taking the "anti-log" of both sides, which is the reverse operation from taking a log.

temperament

When the interval between two pitches corresponds to a whole number ratio of their frequencies, such an interval has a certain stability, or purity, to its sound.  The simpler (i.e. the smaller the numerator and denominator is) the ratio, the better the sound.  If one of those pitches is adjusted slightly in order to meet other requirements of the system, it is said to be tempered.  A musical system created by altering one or more of its constituent intervals from the ideal (generally accepted to be the Just Intonation system) is considered to have a specific temperament.  There have been numerous examples of this throughout history, including Meantone temperament and the famous Well temperament used by J. S. Bach to create his "Well-Tempered Clavier" pieces.  The western arrangement in use today is defined as Equal temperament.

This page was last updated on February 12, 2023

Top Of Page | Home Page | Email Me